×
![]()
neos-574665: Instance-to-Instance Comparison Results
| Type: | Instance |
| Submitter: | NEOS Server Submission |
| Description: | Imported from the MIPLIB2010 submissions. |
| MIPLIB Entry |
Parent Instance (neos-574665)
All other instances below were be compared against this "query" instance.  |
 |
 |
 |
 |
 |
|
Raw
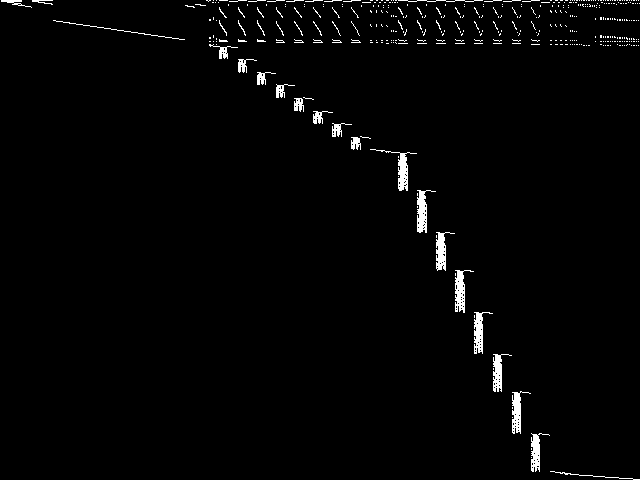
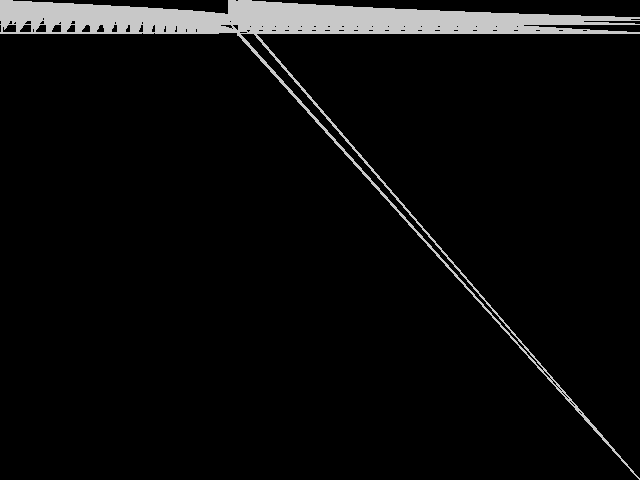
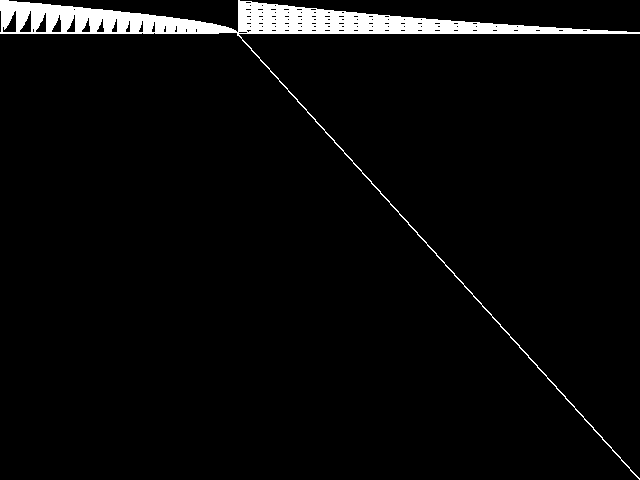
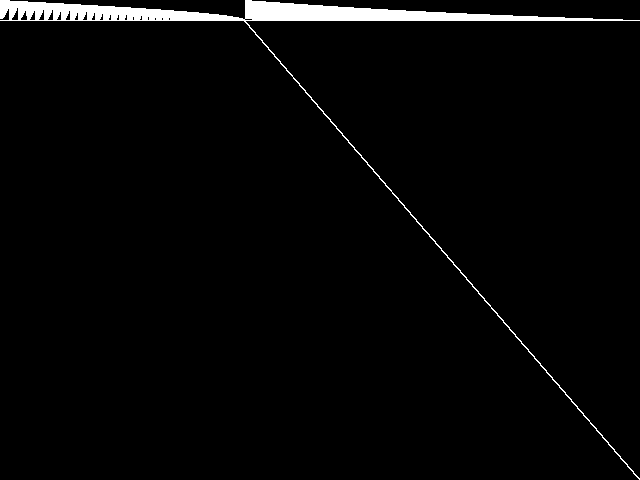
This is the CCM image before the decomposition procedure has been applied.
|
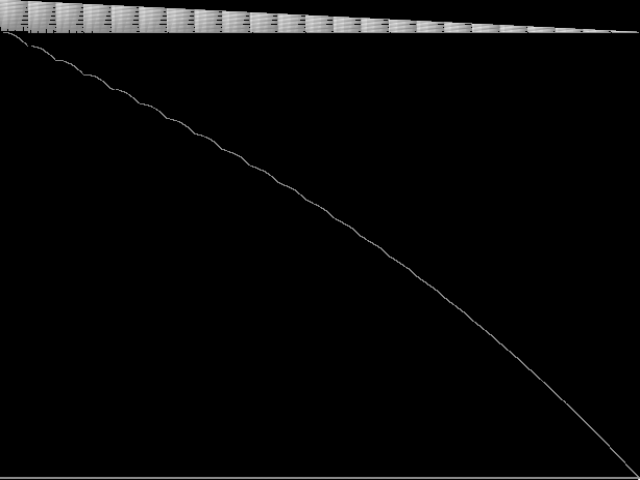
Decomposed
This is the CCM image after a decomposition procedure has been applied. This is the image used by the MIC's image-based comparisons for this query instance.
|
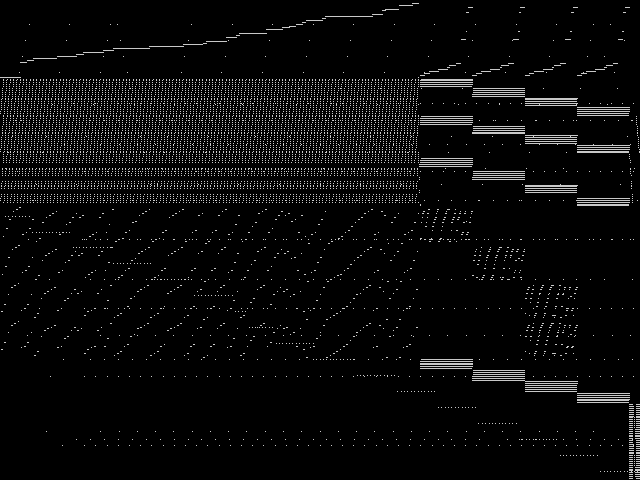
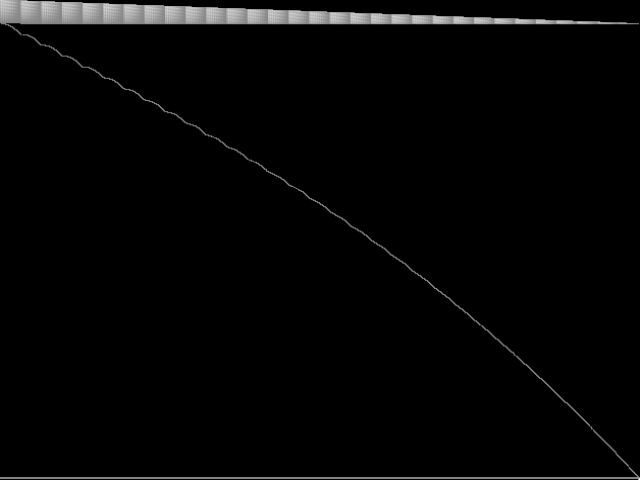
Composite of MIC Top 5
Composite of the five decomposed CCM images from the MIC Top 5.
|
Composite of MIPLIB Top 5
Composite of the five decomposed CCM images from the MIPLIB Top 5.
|
Model Group Composite Image
Composite of the decomposed CCM images for every instance in the same model group as this query.
|
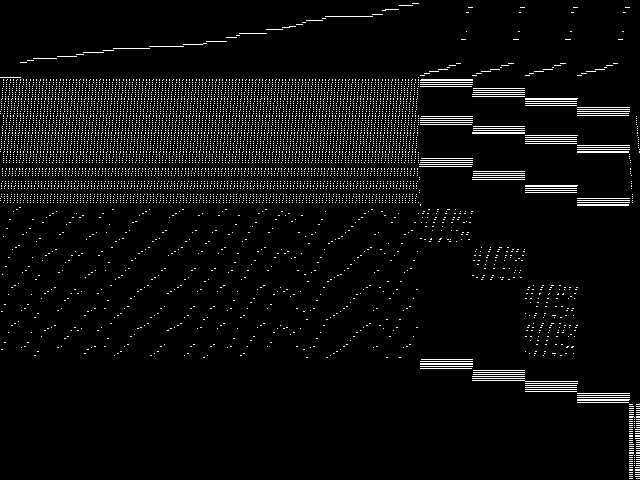
MIC Top 5 Instances
These are the 5 decomposed CCM images that are most similar to decomposed CCM image for the the query instance, according to the ISS metric.  |
Decomposed
These decomposed images were created by GCG.
|
 |
 |
 |
 |
 |
| Name | square23 [MIPLIB] | square31 [MIPLIB] | square37 [MIPLIB] | square41 [MIPLIB] | square47 [MIPLIB] | |
|
Rank / ISS
The image-based structural similarity (ISS) metric measures the Euclidean distance between the image-based feature vectors for the query instance and all other instances. A smaller ISS value indicates greater similarity.
|
1 / 1.013 | 2 / 1.066 | 3 / 1.066 | 4 / 1.066 | 5 / 1.099 | |
|
Raw
These images represent the CCM images in their raw forms (before any decomposition was applied) for the MIC top 5.
|
 |
 |
 |
 |
 |
MIPLIB Top 5 Instances
These are the 5 instances that are most closely related to the query instance, according to the instance statistic-based similarity measure employed by MIPLIB 2017  |
Decomposed
These decomposed images were created by GCG.
|
 |
 |
 |
 |
 |
| Name | gt2 [MIPLIB] | markshare_5_0 [MIPLIB] | markshare_4_0 [MIPLIB] | markshare1 [MIPLIB] | neos-5045105-creuse [MIPLIB] | |
|
Rank / ISS
The image-based structural similarity (ISS) metric measures the Euclidean distance between the image-based feature vectors for the query instance and all model groups. A smaller ISS value indicates greater similarity.
|
87 / 1.394 | 316 / 1.572 | 326 / 1.578 | 362 / 1.601 | 831 / 2.216 | |
|
Raw
These images represent the CCM images in their raw forms (before any decomposition was applied) for the MIPLIB top 5.
|
 |
 |
 |
 |
 |
Instance Summary
The table below contains summary information for neos-574665, the five most similar instances to neos-574665 according to the MIC, and the five most similar instances to neos-574665 according to MIPLIB 2017.
| INSTANCE | SUBMITTER | DESCRIPTION | ISS | RANK | |
|---|---|---|---|---|---|
| Parent Instance | neos-574665 [MIPLIB] | NEOS Server Submission | Imported from the MIPLIB2010 submissions. | 0.000000 | - |
| MIC Top 5 | square23 [MIPLIB] | Sascha Kurz | Squaring the square For a given integer n, determine the minimum number of squares in a tiling of an \\(n\\times n\\) square using using only integer sided squares of smaller size. (Although the models get quite large even for moderate n, they can be solved to optimality for all \\(n \\le 61\\), while challenging the MIP solver, especially the presolver.) | 1.013300 | 1 |
| square31 [MIPLIB] | Sascha Kurz | Squaring the square For a given integer n, determine the minimum number of squares in a tiling of an \\(n\\times n\\) square using using only integer sided squares of smaller size. (Although the models get quite large even for moderate n, they can be solved to optimality for all \\(n \\le 61\\), while challenging the MIP solver, especially the presolver.) | 1.065604 | 2 | |
| square37 [MIPLIB] | Sascha Kurz | Squaring the square For a given integer n, determine the minimum number of squares in a tiling of an \\(n\\times n\\) square using using only integer sided squares of smaller size. (Although the models get quite large even for moderate n, they can be solved to optimality for all \\(n \\le 61\\), while challenging the MIP solver, especially the presolver.) | 1.066362 | 3 | |
| square41 [MIPLIB] | Sascha Kurz | Squaring the square For a given integer n, determine the minimum number of squares in a tiling of an \\(n\\times n\\) square using using only integer sided squares of smaller size. (Although the models get quite large even for moderate n, they can be solved to optimality for all \\(n \\le 61\\), while challenging the MIP solver, especially the presolver.) | 1.066443 | 4 | |
| square47 [MIPLIB] | Sascha Kurz | Squaring the square For a given integer n, determine the minimum number of squares in a tiling of an \\(n\\times n\\) square using using only integer sided squares of smaller size. (Although the models get quite large even for moderate n, they can be solved to optimality for all \\(n \\le 61\\), while challenging the MIP solver, especially the presolver.) | 1.099451 | 5 | |
| MIPLIB Top 5 | gt2 [MIPLIB] | MIPLIB submission pool | Imported from the MIPLIB2010 submissions. | 1.393625 | 87 |
| markshare_5_0 [MIPLIB] | G. Cornuéjols, M. Dawande | Market sharing problem | 1.572032 | 316 | |
| markshare_4_0 [MIPLIB] | G. Cornuéjols, M. Dawande | Market sharing problem | 1.578101 | 326 | |
| markshare1 [MIPLIB] | G. Cornuéjols, M. Dawande | Market sharing problem | 1.601111 | 362 | |
| neos-5045105-creuse [MIPLIB] | Jeff Linderoth | (None provided) | 2.215512 | 831 |
neos-574665: Instance-to-Model Comparison Results
| Model Group Assignment from MIPLIB: | neos-pseudoapplication-78 |
| Assigned Model Group Rank/ISS in the MIC: | 199 / 3.558 |
MIC Top 5 Model Groups
These are the 5 model group composite (MGC) images that are most similar to the decomposed CCM image for the query instance, according to the ISS metric.  |
These are model group composite (MGC) images for the MIC top 5 model groups.
|
 |
 |
 |
 |
 |
| Name | square | supportvectormachine | noip | neos-pseudoapplication-74 | drayage | |
|
Rank / ISS
The image-based structural similarity (ISS) metric measures the Euclidean distance between the image-based feature vectors for the query instance and all other instances. A smaller ISS value indicates greater similarity.
|
1 / 1.166 | 2 / 1.668 | 3 / 1.730 | 4 / 1.757 | 5 / 1.764 |
Model Group Summary
The table below contains summary information for the five most similar model groups to neos-574665 according to the MIC.
| MODEL GROUP | SUBMITTER | DESCRIPTION | ISS | RANK | |
|---|---|---|---|---|---|
| MIC Top 5 | square | Sascha Kurz | Squaring the square For a given integer n, determine the minimum number of squares in a tiling of an \\(n\\times n\\) square using using only integer sided squares of smaller size. (Although the models get quite large even for moderate n, they can be solved to optimality for all \\(n \\le 61\\), while challenging the MIP solver, especially the presolver.) | 1.165579 | 1 |
| supportvectormachine | Toni Sorrell | Suport vector machine with ramp loss. GSVM2-RL is the formulation found in Hess E. and Brooks P. (2015) paper, The Support Vector Machine and Mixed Integer Linear Programming: Ramp Loss SVM with L1-Norm Regularization | 1.667876 | 2 | |
| noip | Christopher Hojny | integer programming formulation that verifies that no integer programming formulation of a given 0/1-point set exists | 1.729583 | 3 | |
| neos-pseudoapplication-74 | Jeff Linderoth | (None provided) | 1.757116 | 4 | |
| drayage | F. Jordan Srour | The .rar file contains three folders: 1) R_mps with all of the models (165, organized into 5 groups R0_, R25_, R50_, R75_, and R100_*), 2) results_and_runtimes with datafiles on the runtime and results, and 3) doc with documentation on the models in the form of a pdf. | 1.764464 | 5 |

