×
![]()
supportvectormachine
| Type: | Model Group |
| Submitter: | Toni Sorrell |
| Description: | Suport vector machine with ramp loss. GSVM2-RL is the formulation found in Hess E. and Brooks P. (2015) paper, The Support Vector Machine and Mixed Integer Linear Programming: Ramp Loss SVM with L1-Norm Regularization |
Parent Model Group (supportvectormachine)
All other model groups below were be compared against this "query" model group.  |
 |
|
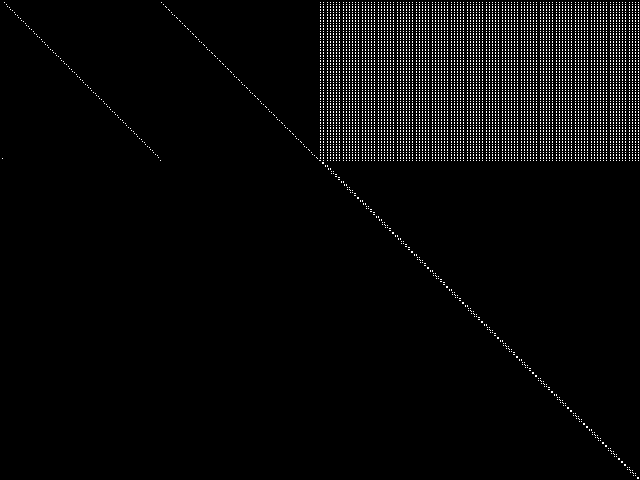
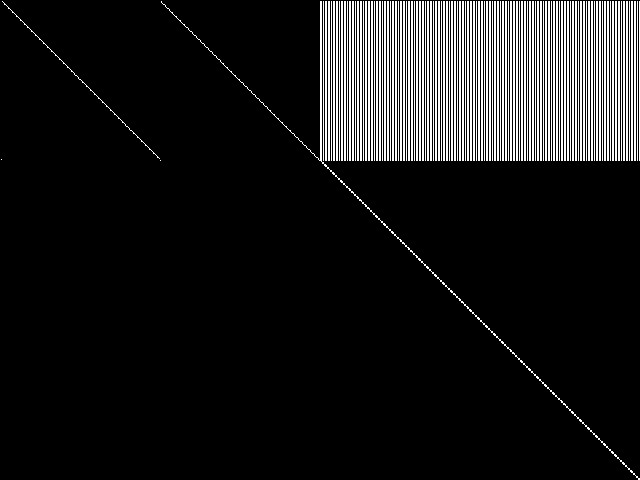
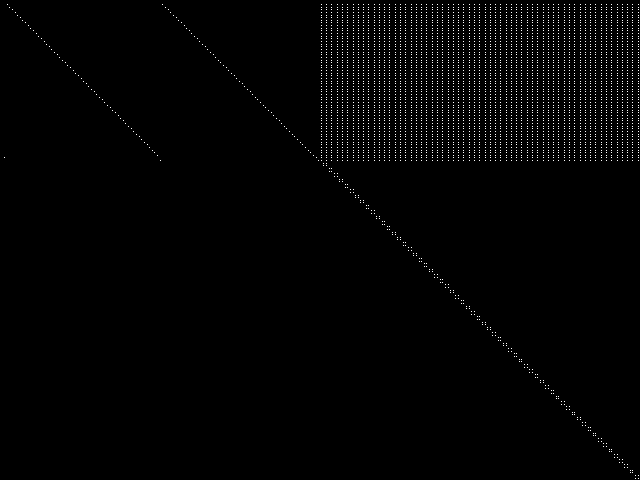
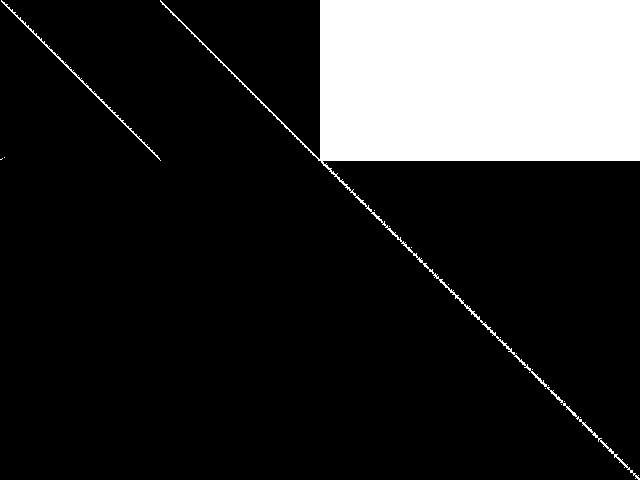
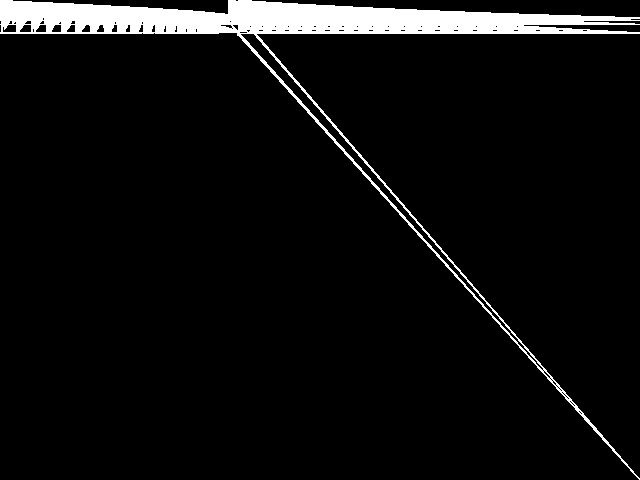
Model Group Composite (MGC) image
Composite of the decomposed CCM images for every instance in the query model group.
|
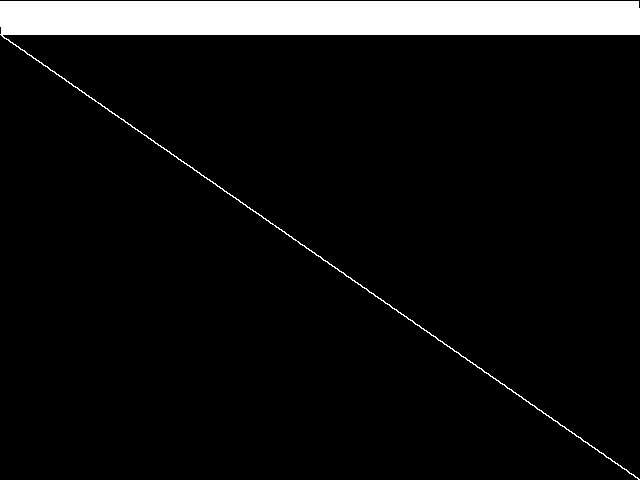
Component Instances (Decomposed)
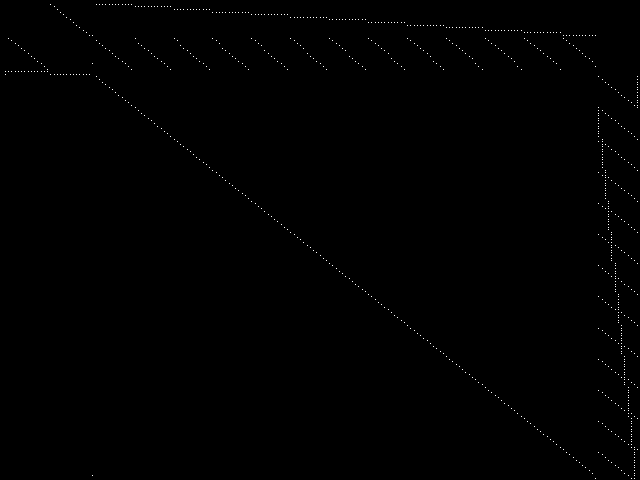
These are the decomposed CCM images for each instance in the query model group.MIC Top 5 Model Groups
These are the 5 MGC images that are most similar to the MGC image for the query model group, according to the ISS metric.  |
FIXME - These are model group composite images.
|
 |
 |
 |
 |
 |
| Name | square | neos-pseudoapplication-74 | scp | neos-pseudoapplication-2 | neos-pseudoapplication-109 | |
|
Rank / ISS
The image-based structural similarity (ISS) metric measures the Euclidean distance between the image-based feature vectors for the query model group and all other model groups. A smaller ISS value indicates greater similarity.
|
1 / 1.397 | 2 / 1.491 | 3 / 1.509 | 4 / 1.517 | 5 / 1.550 |
Model Group Summary
The table below contains summary information for supportvectormachine, and for the five most similar model groups to supportvectormachine according to the MIC.
| MODEL GROUP | SUBMITTER | DESCRIPTION | ISS | RANK | |
|---|---|---|---|---|---|
| Parent Model Group | supportvectormachine | Toni Sorrell | Suport vector machine with ramp loss. GSVM2-RL is the formulation found in Hess E. and Brooks P. (2015) paper, The Support Vector Machine and Mixed Integer Linear Programming: Ramp Loss SVM with L1-Norm Regularization | 0.000000 | - |
| MIC Top 5 | square | Sascha Kurz | Squaring the square For a given integer n, determine the minimum number of squares in a tiling of an \\(n\\times n\\) square using using only integer sided squares of smaller size. (Although the models get quite large even for moderate n, they can be solved to optimality for all \\(n \\le 61\\), while challenging the MIP solver, especially the presolver.) | 1.396914 | 1 |
| neos-pseudoapplication-74 | Jeff Linderoth | (None provided) | 1.490794 | 2 | |
| scp | Shunji Umetani | This is a random test model generator for SCP using the scheme of the following paper, namely the column cost c[j] are integer randomly generated from [1,100]; every column covers at least one row; and every row is covered by at least two columns. see reference: E. Balas and A. Ho, Set covering algorithms using cutting planes, heuristics, and subgradient optimization: A computational study, Mathematical Programming, 12 (1980), 37-60. We have newly generated Classes I-N with the following parameter values, where each class has five models. We have also generated reduced models by a standard pricing method in the following paper: S. Umetani and M. Yagiura, Relaxation heuristics for the set covering problem, Journal of the Operations Research Society of Japan, 50 (2007), 350-375. You can obtain the model generator program from the following web site. https://sites.google.com/site/shunjiumetani/benchmark | 1.508844 | 3 | |
| neos-pseudoapplication-2 | NEOS Server Submission | Imported from the MIPLIB2010 submissions. | 1.516950 | 4 | |
| neos-pseudoapplication-109 | Jeff Linderoth | (None provided) | 1.550048 | 5 |