Instance - t1717

t1717: Instance-to-Instance Comparison Results

Type:	Instance
Submitter:	R. Borndörfer
Description:	Vehicle scheduling set partitioning problem from Berlin's Telebus handicapped people's transportation system
	MIPLIB Entry


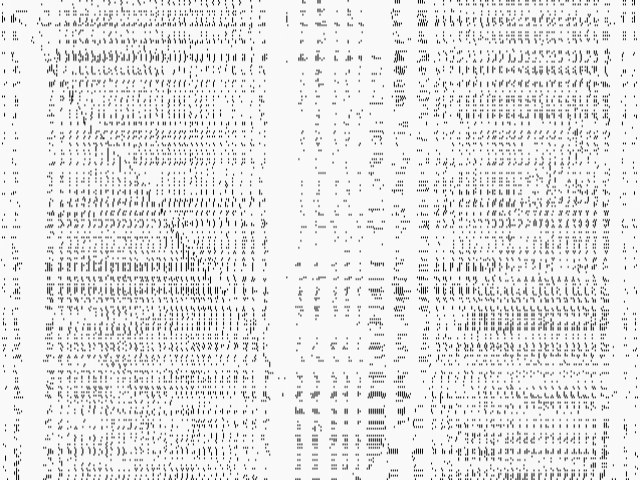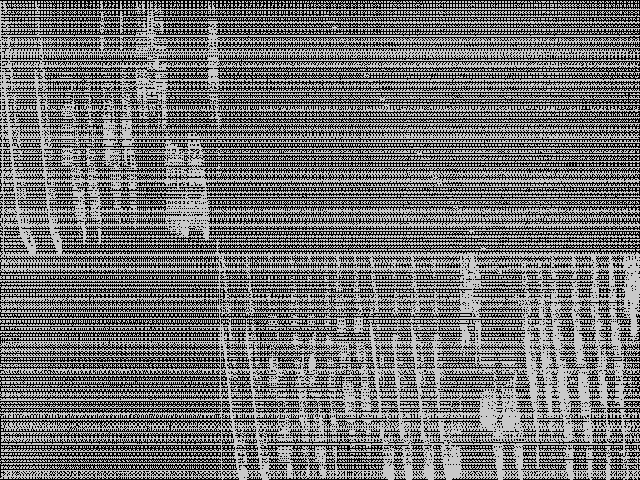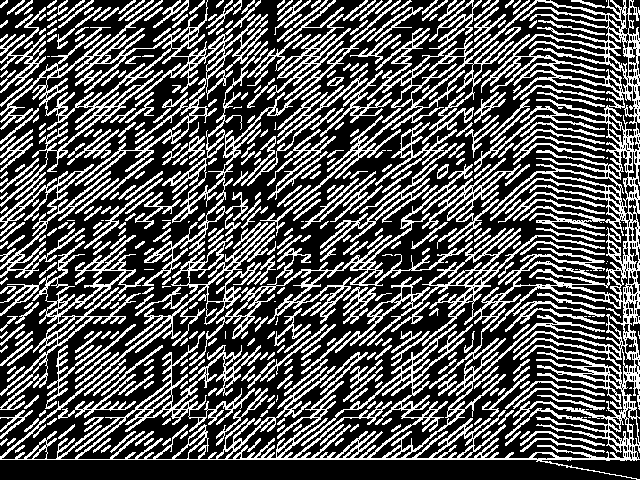
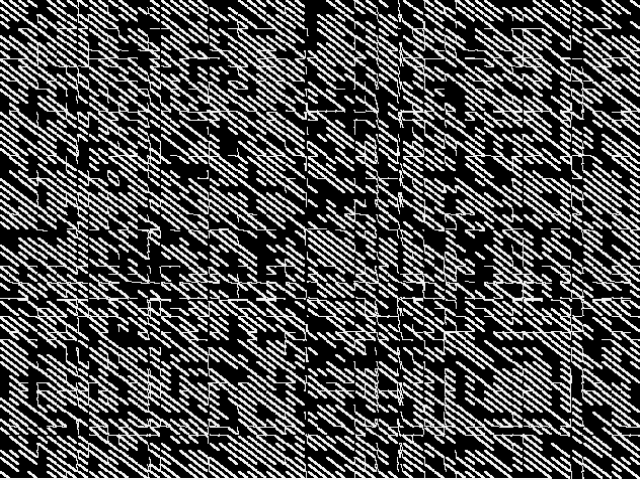
	Raw This is the CCM image before the decomposition procedure has been applied.	Decomposed This is the CCM image after a decomposition procedure has been applied. This is the image used by the MIC's image-based comparisons for this query instance.	Composite of MIC Top 5 Composite of the five decomposed CCM images from the MIC Top 5.	Composite of MIPLIB Top 5 Composite of the five decomposed CCM images from the MIPLIB Top 5.	Model Group Composite Image Composite of the decomposed CCM images for every instance in the same model group as this query.

Decomposed These decomposed images were created by GCG.
Name	neos-4760493-puerua [MIPLIB]	ex10 [MIPLIB]	sorrell7 [MIPLIB]	t1722 [MIPLIB]	dc1l [MIPLIB]
Rank / ISS The image-based structural similarity (ISS) metric measures the Euclidean distance between the image-based feature vectors for the query instance and all other instances. A smaller ISS value indicates greater similarity.	1 / 0.762	2 / 0.791	3 / 0.829	4 / 0.843	5 / 0.899
Raw These images represent the CCM images in their raw forms (before any decomposition was applied) for the MIC top 5.

Decomposed These decomposed images were created by GCG.
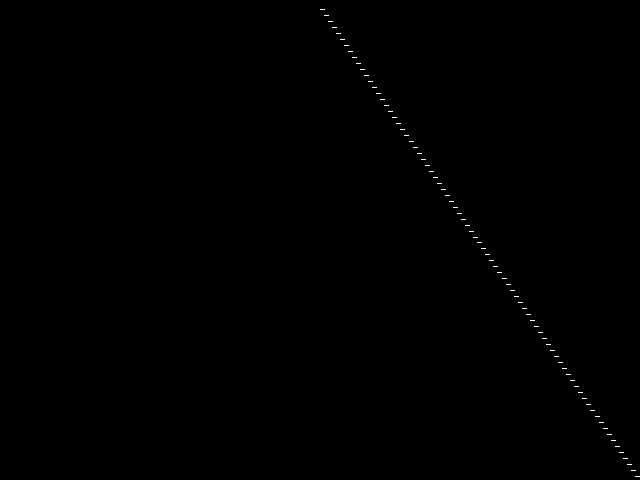Name	t1722 [MIPLIB]	eilC76-2 [MIPLIB]	eil33-2 [MIPLIB]	ds [MIPLIB]	h80x6320 [MIPLIB]
Rank / ISS The image-based structural similarity (ISS) metric measures the Euclidean distance between the image-based feature vectors for the query instance and all model groups. A smaller ISS value indicates greater similarity.	4 / 0.843	30 / 1.387	55 / 1.862	208 / 2.898	990 / 3.938
Raw These images represent the CCM images in their raw forms (before any decomposition was applied) for the MIPLIB top 5.

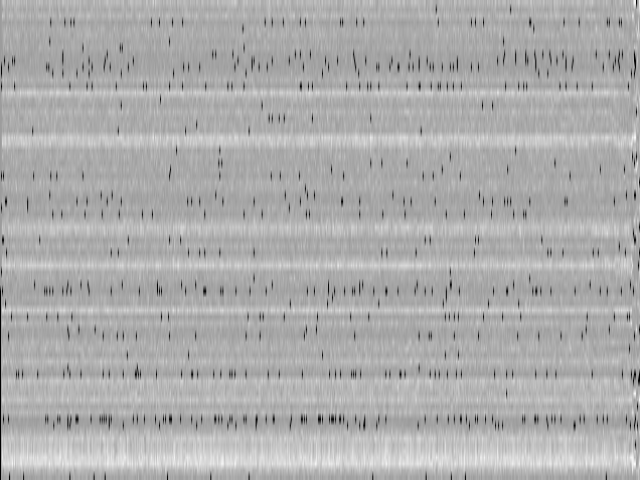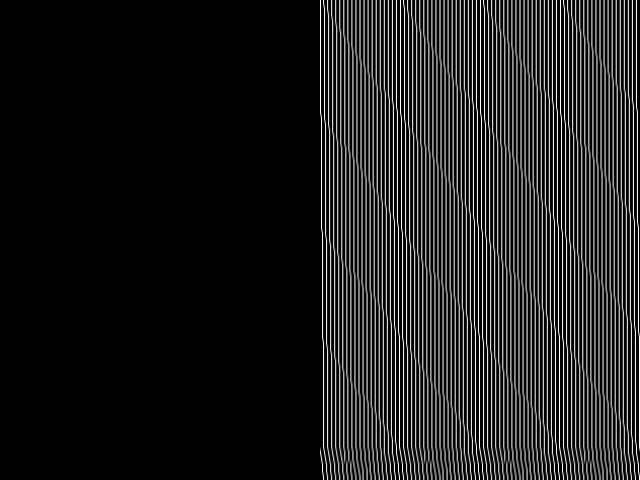Instance Summary

The table below contains summary information for t1717, the five most similar instances to t1717 according to the MIC, and the five most similar instances to t1717 according to MIPLIB 2017.

	INSTANCE	SUBMITTER	DESCRIPTION	ISS	RANK
Parent Instance	t1717 [MIPLIB]	R. Borndörfer	Vehicle scheduling set partitioning problem from Berlin's Telebus handicapped people's transportation system	0.000000	-
MIC Top 5	neos-4760493-puerua [MIPLIB]	Hans Mittelmann	Collection of anonymous submissions to the NEOS Server for Optimization	0.762355	1
	ex10 [MIPLIB]	Iulian Ober	Formulations of Boolean SAT instance	0.790762	2
	sorrell7 [MIPLIB]	Toni Sorrell	These instances are based on Neil Sloane's Challenge problems: Independent Sets in Graphs.	0.829031	3
	t1722 [MIPLIB]	R. Borndörfer	Vehicle scheduling set partitioning problem from Berlin's Telebus handicapped people's transportation system	0.843422	4
	dc1l [MIPLIB]	Double-Click SAS	Crew scheduling instance	0.899164	5
MIPLIB Top 5	t1722 [MIPLIB]	R. Borndörfer	Vehicle scheduling set partitioning problem from Berlin's Telebus handicapped people's transportation system	0.843422	4
	eilC76-2 [MIPLIB]	J. Linderoth	Set partitioning problem approximation for capicated vehicle routing problem instance from TSPLIB Imported from the MIPLIB2010 submissions.	1.386990	30
	eil33-2 [MIPLIB]	J. Linderoth	Set partitioning problem approximation for capicated vehicle routing problem instance from TSPLIB	1.862428	55
	ds [MIPLIB]	MIPLIB submission pool	Imported from MIPLIB2003.	2.898276	208
	h80x6320 [MIPLIB]	MIPLIB submission pool	Imported from the MIPLIB2010 submissions.	3.937552	990

t1717: Instance-to-Model Comparison Results

Model Group Assignment from MIPLIB:	no model group assignment
Assigned Model Group Rank/ISS in the MIC:	N.A. / N.A.

These are model group composite (MGC) images for the MIC top 5 model groups.
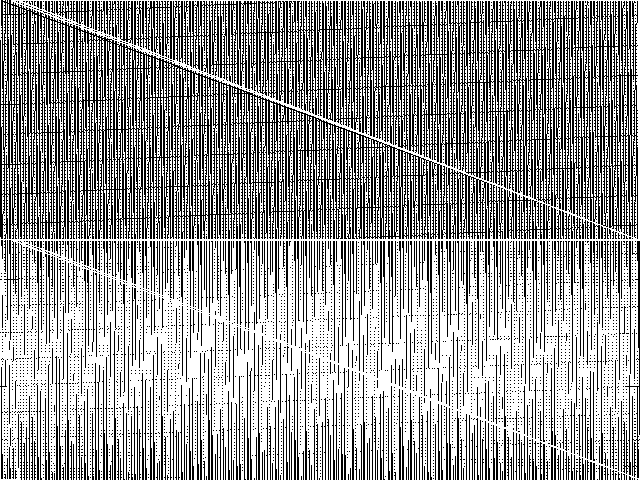Name	neos-pseudoapplication-106	iis	air	neos-pseudoapplication-23	2hopcds
Rank / ISS The image-based structural similarity (ISS) metric measures the Euclidean distance between the image-based feature vectors for the query instance and all other instances. A smaller ISS value indicates greater similarity.	1 / 0.725	2 / 0.796	3 / 1.054	4 / 1.087	5 / 1.156

Model Group Summary

The table below contains summary information for the five most similar model groups to t1717 according to the MIC.

	MODEL GROUP	SUBMITTER	DESCRIPTION	ISS	RANK
MIC Top 5	neos-pseudoapplication-106	Hans Mittelmann	Collection of anonymous submissions to the NEOS Server for Optimization	0.724963	1
	iis	Marc Pfetsch	23 "middlehard" Set-Covering Models for MIPLIB: they have a small number of variables compared to the number of constraints and CPLEX 12.1 needs about one hour to solve them.For more information, have a look into the readme file which explains how the models can be created.	0.796093	2
	air	G. Astfalk	Airline crew scheduling set partitioning problem	1.053631	3
	neos-pseudoapplication-23	NEOS Server Submission	Imported from the MIPLIB2010 submissions.	1.087221	4
	2hopcds	Austin Buchanan	A problem in wireless networks. The objective is to select a minimum number of relay nodes so that any two nonadjacent nodes can communicate by way of the chosen relay nodes in at most s hops, where s is a problem input. The 2-hop case of this problem can be formulated as a set cover/hitting set problem with n binary variables and n^2 constraints: _{ k N(i) N(j) } x_k 1 for nonadjacent node pairs {i,j}. Despite the formulation's simplicity, models with as few as 120 variables are left unsolved after one hour using Gurobi 7.0.2.	1.155842	5

t1717: Instance-to-Instance Comparison Results

Parent Instance (t1717)

MIC Top 5 Instances

MIPLIB Top 5 Instances

Instance Summary

t1717: Instance-to-Model Comparison Results

MIC Top 5 Model Groups

Model Group Summary