×
![]()
mitre: Instance-to-Instance Comparison Results
| Type: | Instance |
| Submitter: | MIPLIB submission pool |
| Description: | Imported from the MIPLIB2010 submissions. |
| MIPLIB Entry |
Parent Instance (mitre)
All other instances below were be compared against this "query" instance.  |
 |
 |
 |
 |
 |
|
Raw
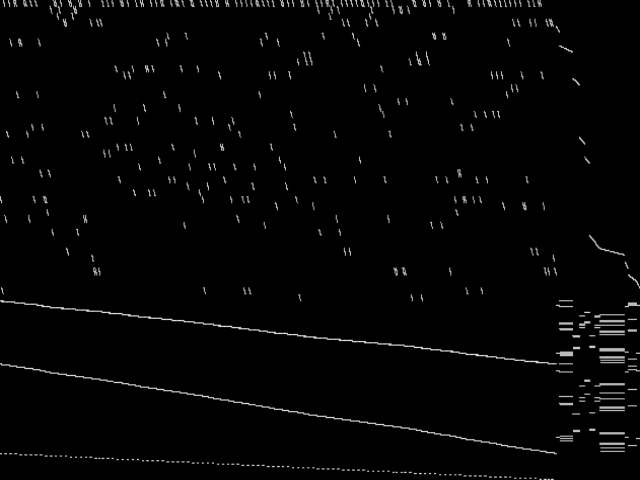
This is the CCM image before the decomposition procedure has been applied.
|
Decomposed
This is the CCM image after a decomposition procedure has been applied. This is the image used by the MIC's image-based comparisons for this query instance.
|
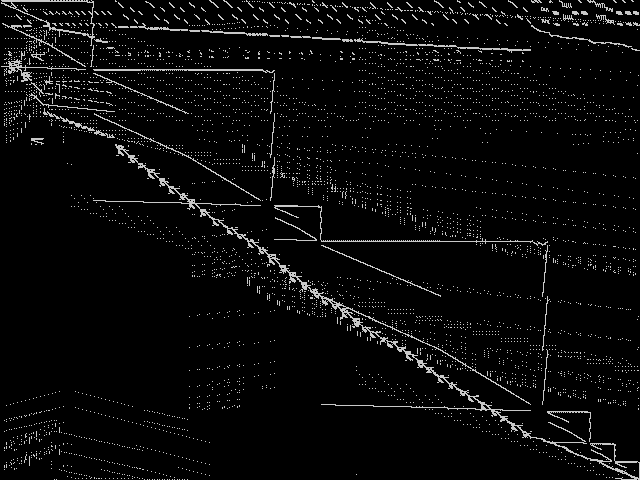
Composite of MIC Top 5
Composite of the five decomposed CCM images from the MIC Top 5.
|
Composite of MIPLIB Top 5
Composite of the five decomposed CCM images from the MIPLIB Top 5.
|
Model Group Composite Image
Composite of the decomposed CCM images for every instance in the same model group as this query.
|
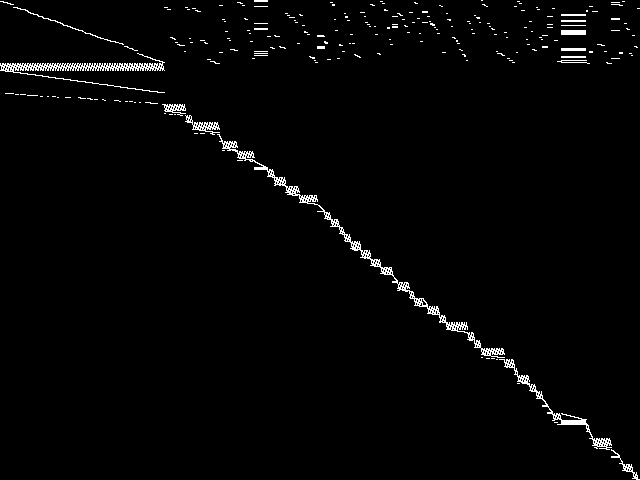
MIC Top 5 Instances
These are the 5 decomposed CCM images that are most similar to decomposed CCM image for the the query instance, according to the ISS metric.  |
Decomposed
These decomposed images were created by GCG.
|
 |
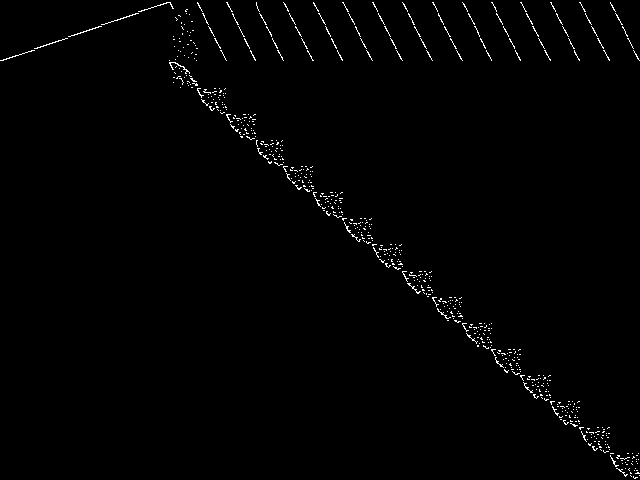 |
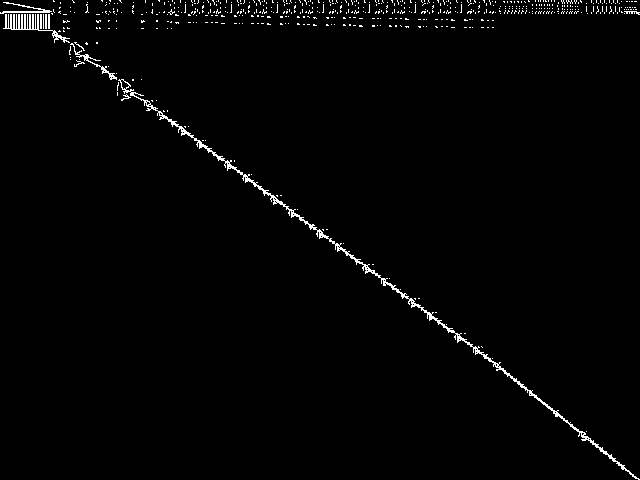 |
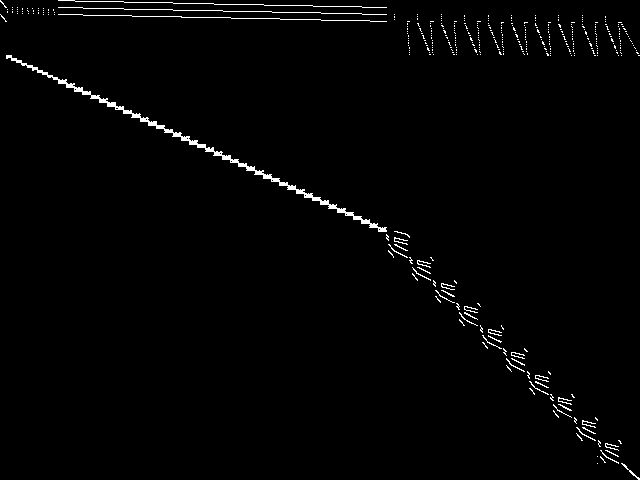 |
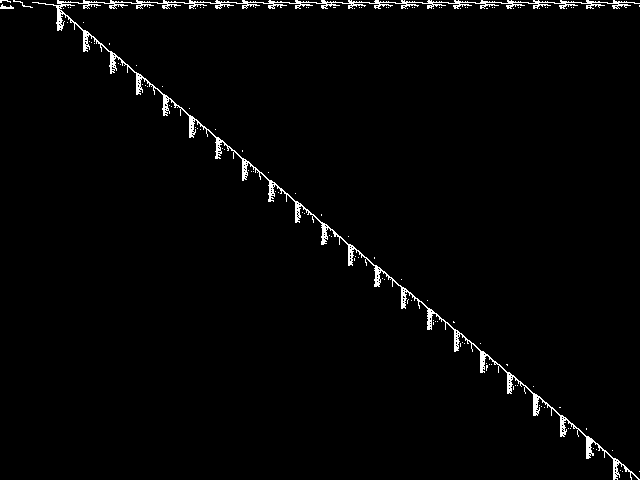 |
| Name | umts [MIPLIB] | ta1-UUM [MIPLIB] | mappingmesh3x3mpeg2i [MIPLIB] | neos-3762025-ognon [MIPLIB] | fastxgemm-n3r22s4t6 [MIPLIB] | |
|
Rank / ISS
The image-based structural similarity (ISS) metric measures the Euclidean distance between the image-based feature vectors for the query instance and all other instances. A smaller ISS value indicates greater similarity.
|
1 / 0.862 | 2 / 0.865 | 3 / 0.882 | 4 / 0.912 | 5 / 0.923 | |
|
Raw
These images represent the CCM images in their raw forms (before any decomposition was applied) for the MIC top 5.
|
 |
 |
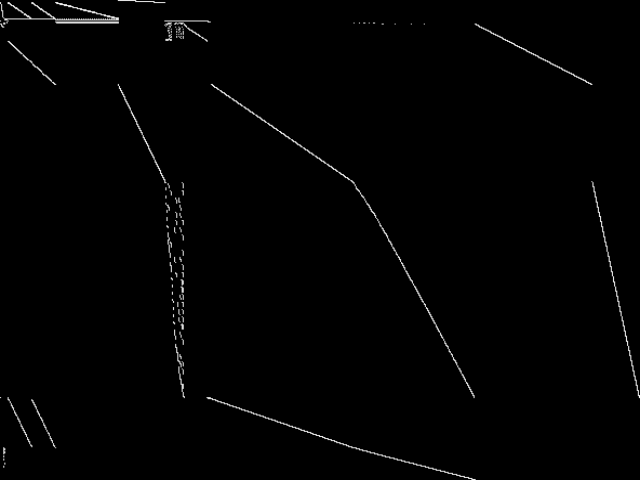 |
 |
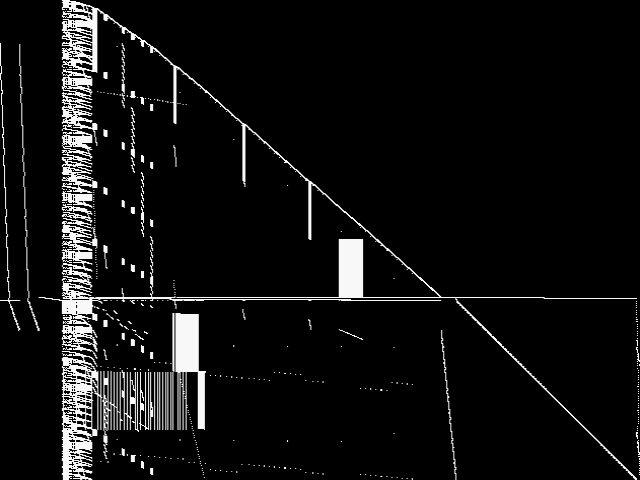 |
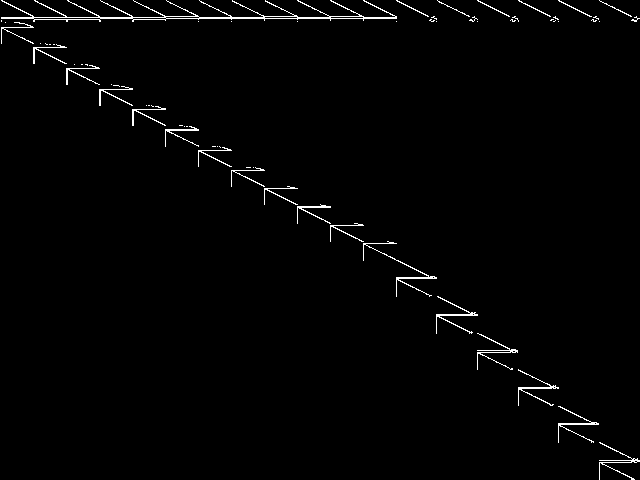
MIPLIB Top 5 Instances
These are the 5 instances that are most closely related to the query instance, according to the instance statistic-based similarity measure employed by MIPLIB 2017  |
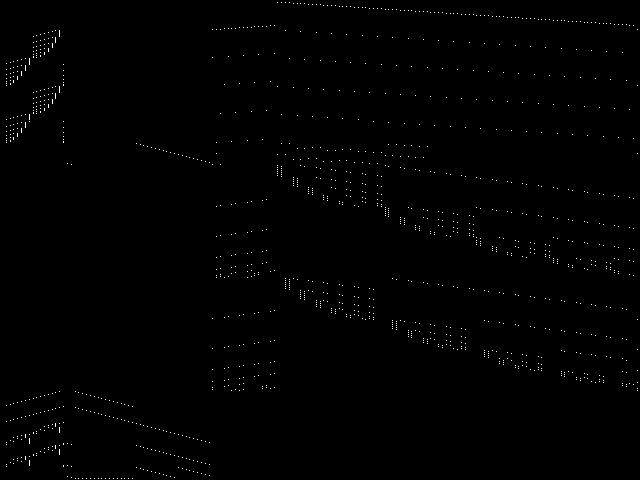
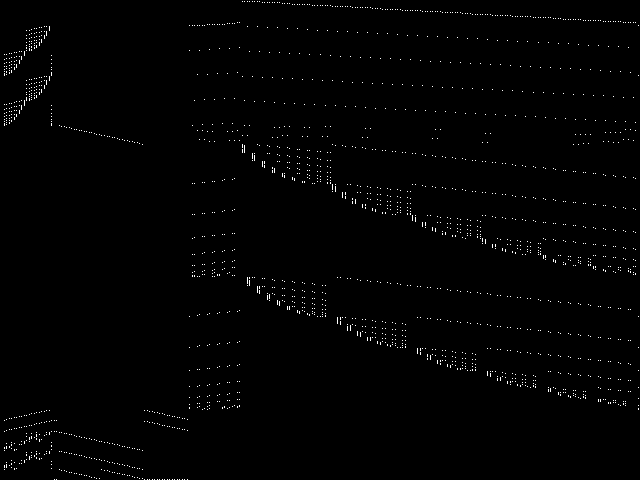
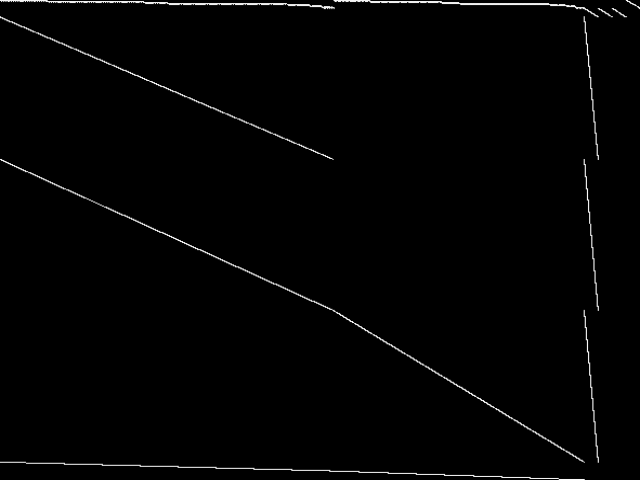
Decomposed
These decomposed images were created by GCG.
|
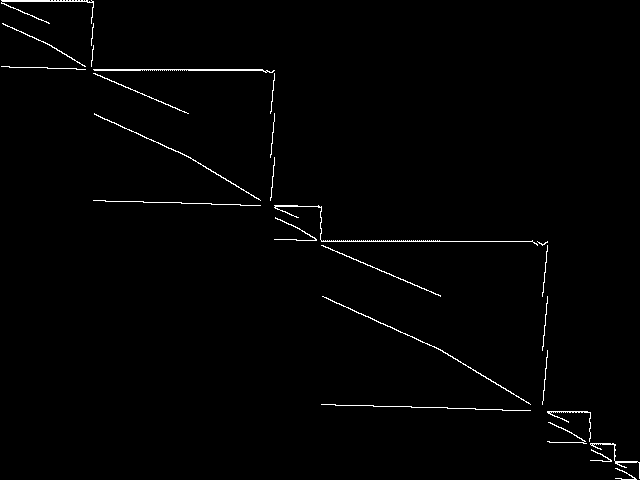 |
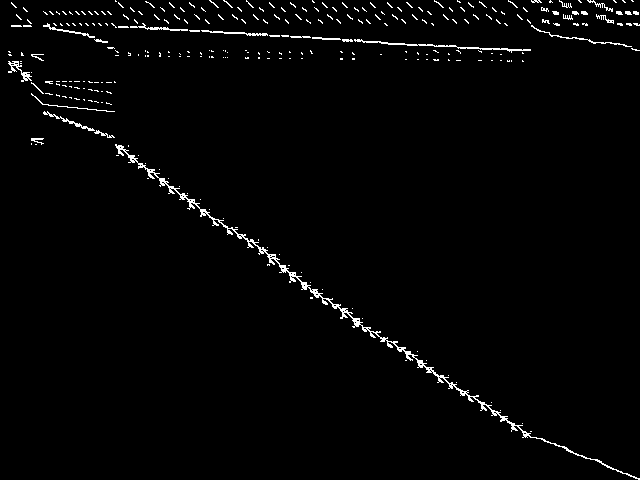 |
 |
 |
 |
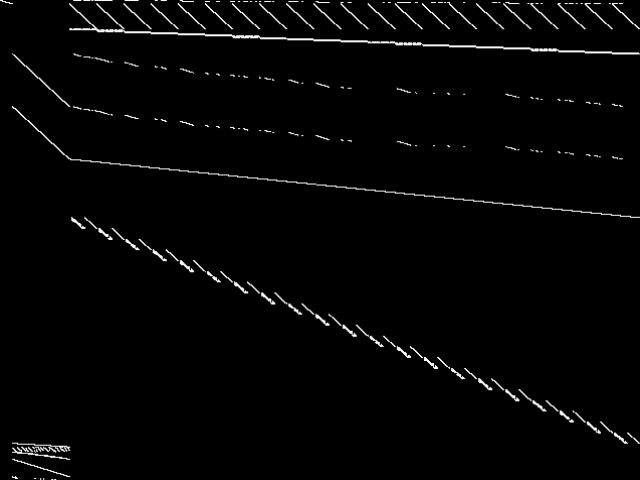
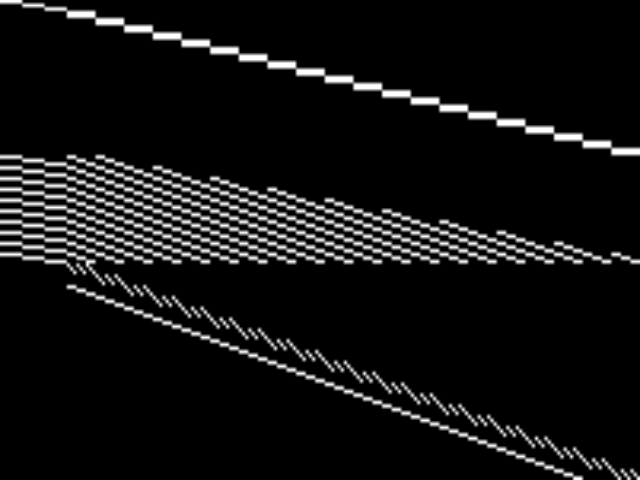
| Name | neos-3696678-lyvia [MIPLIB] | neos-932721 [MIPLIB] | p0201 [MIPLIB] | graphdraw-gemcutter [MIPLIB] | graphdraw-domain [MIPLIB] | |
|
Rank / ISS
The image-based structural similarity (ISS) metric measures the Euclidean distance between the image-based feature vectors for the query instance and all model groups. A smaller ISS value indicates greater similarity.
|
257 / 1.322 | 309 / 1.394 | 323 / 1.413 | 400 / 1.494 | 458 / 1.544 | |
|
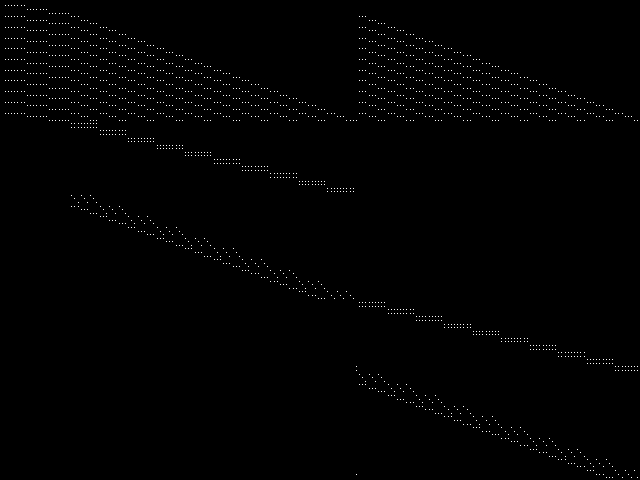
Raw
These images represent the CCM images in their raw forms (before any decomposition was applied) for the MIPLIB top 5.
|
 |
 |
 |
 |
 |
Instance Summary
The table below contains summary information for mitre, the five most similar instances to mitre according to the MIC, and the five most similar instances to mitre according to MIPLIB 2017.
| INSTANCE | SUBMITTER | DESCRIPTION | ISS | RANK | |
|---|---|---|---|---|---|
| Parent Instance | mitre [MIPLIB] | MIPLIB submission pool | Imported from the MIPLIB2010 submissions. | 0.000000 | - |
| MIC Top 5 | umts [MIPLIB] | C. Polo | Telecommunications network model | 0.861519 | 1 |
| ta1-UUM [MIPLIB] | MIPLIB submission pool | Imported from the MIPLIB2010 submissions. | 0.864982 | 2 | |
| mappingmesh3x3mpeg2i [MIPLIB] | Gleb Belov | These are the instances from MiniZinc Challenges 2012-2016 (see www.minizinc.org), compiled for MIP WITH INDICATOR CONSTRAINTS using the develop branch of MiniZinc and CPLEX 12.7.1 on 30 April 2017. Thus, these instances can only be handled by solvers accepting indicator constraints. For instances compiled with big-M/domain decomposition only, see my previous submission to MIPLIB.To recompile, create a directory MODELS, a list lst12_16.txt of the instances with full paths to mzn/dzn files of each instance per line, and say$> ~/install/libmzn/tests/benchmarking/mzn-test.py -l ../lst12_16.txt -slvPrf MZN-CPLEX -debug 1 -addOption "-timeout 3 -D fIndConstr=true -D fMIPdomains=false" -useJoinedName "-writeModel MODELS_IND/%s.mps" Alternatively, you can compile individual instance as follows: $> mzn-cplex -v -s -G linear -output-time ../challenge_2012_2016/mznc2016_probs/zephyrus/zephyrus.mzn ../challenge_2012_2016/mznc2016_p/zephyrus/14__8__6__3.dzn -a -timeout 3 -D fIndConstr=true -D fMIPdomains=false -writeModel MODELS_IND/challenge_2012_2016mznc2016_probszephyruszephyrusmzn-challenge_2012_2016mznc2016_probszephyrus14__8__6__3dzn.mps | 0.882407 | 3 | |
| neos-3762025-ognon [MIPLIB] | Jeff Linderoth | (None provided) | 0.911721 | 4 | |
| fastxgemm-n3r22s4t6 [MIPLIB] | Laurent Sorber | Naive multiplication of two N by N matrices requires N^3 scalar multiplications. For N=2, Strassen showed that it could be done in only R=7 < 8=N^3 multiplications. For N=3, it is known that 19 <= R <= 23, and for N=4 it is known that 34 <= R <= 49. This repository contains code that generates a mixed-integer linear program (MILP) formulation of the fast matrix multiplication problem for finding solutions with R < N^3 and proving that they are optimal. For a more detailed description, see the accompanying manuscript. | 0.922782 | 5 | |
| MIPLIB Top 5 | neos-3696678-lyvia [MIPLIB] | Jeff Linderoth | (None provided) | 1.321933 | 257 |
| neos-932721 [MIPLIB] | NEOS Server Submission | Imported from the MIPLIB2010 submissions. | 1.393748 | 309 | |
| p0201 [MIPLIB] | MIPLIB submission pool | Imported from the MIPLIB2010 submissions. | 1.413433 | 323 | |
| graphdraw-gemcutter [MIPLIB] | Cézar Augusto Nascimento e Silva | In the Graph Drawing problem a set of symbols must be placed in a plane and their connections routed. The objective is to produce aesthetically pleasant, easy to read diagrams. As a primary concern one usually tries to minimize edges crossing, edges' length, waste of space and number of bents in the connections. When formulated with these constraints the problem becomes NP-Hard . In practice many additional complicating requirements can be included, such as non-uniform sizes for symbols. Thus, some heuristics such as the generalized force-direct method and Simulated Annealing have been proposed to tackle this problem. uses a grid structure to approach the Entity-Relationship (ER) drawing problem, emphasizing the differences between ER drawing and the more classical circuit drawing problems. presented different ways of producing graph layouts (e.g.: tree, orthogonal, visibility representations, hierarchic, among others) for general graphs with applications on different subjects. The ability to automatically produce high quality layouts is very important in many applications, one of these is Software Engineering: the availability of easy to understand ER diagrams, for instance, can improve the time needed for developers to master database models and increase their productivity. Our solution approach involves two phases: (\\(i\\)) firstly the optimal placement of entities is solved, i.e.: entities are positioned so as to minimize the distances between connected entities; and (\\(ii\\)) secondly, edges are routed minimizing bends and avoiding the inclusion of connectors too close. We present the model for the first phase of our problem. | 1.493723 | 400 | |
| graphdraw-domain [MIPLIB] | Cézar Augusto Nascimento e Silva | In the Graph Drawing problem a set of symbols must be placed in a plane and their connections routed. The objective is to produce aesthetically pleasant, easy to read diagrams. As a primary concern one usually tries to minimize edges crossing, edges' length, waste of space and number of bents in the connections. When formulated with these constraints the problem becomes NP-Hard . In practice many additional complicating requirements can be included, such as non-uniform sizes for symbols. Thus, some heuristics such as the generalized force-direct method and Simulated Annealing have been proposed to tackle this problem. uses a grid structure to approach the Entity-Relationship (ER) drawing problem, emphasizing the differences between ER drawing and the more classical circuit drawing problems. presented different ways of producing graph layouts (e.g.: tree, orthogonal, visibility representations, hierarchic, among others) for general graphs with applications on different subjects. The ability to automatically produce high quality layouts is very important in many applications, one of these is Software Engineering: the availability of easy to understand ER diagrams, for instance, can improve the time needed for developers to master database models and increase their productivity. Our solution approach involves two phases: (\\(i\\)) firstly the optimal placement of entities is solved, i.e.: entities are positioned so as to minimize the distances between connected entities; and (\\(ii\\)) secondly, edges are routed minimizing bends and avoiding the inclusion of connectors too close. We present the model for the first phase of our problem. | 1.543505 | 458 |
mitre: Instance-to-Model Comparison Results
| Model Group Assignment from MIPLIB: | no model group assignment |
| Assigned Model Group Rank/ISS in the MIC: | N.A. / N.A. |
MIC Top 5 Model Groups
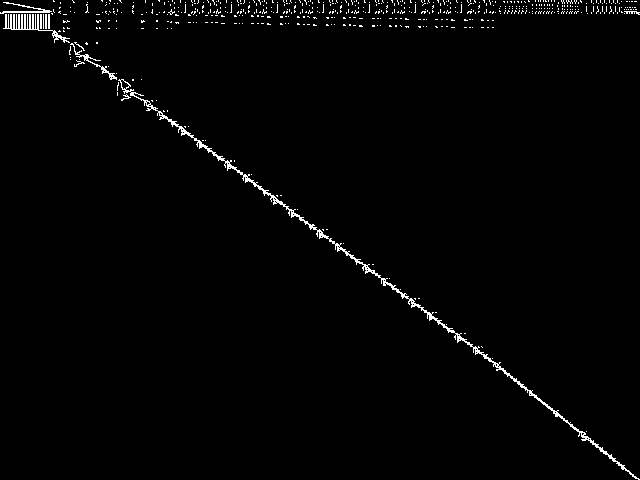
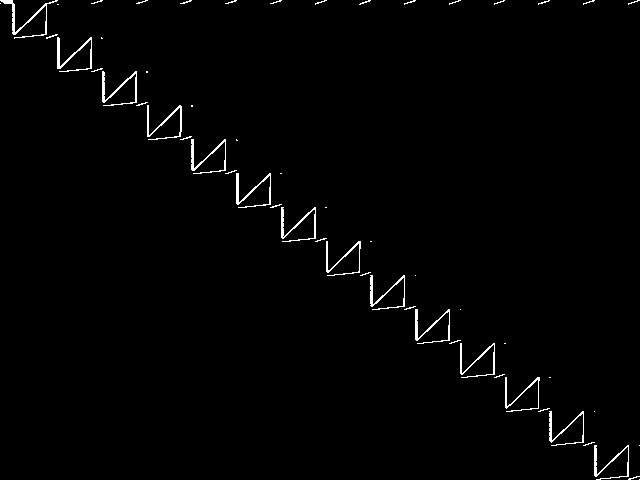
These are the 5 model group composite (MGC) images that are most similar to the decomposed CCM image for the query instance, according to the ISS metric.  |
These are model group composite (MGC) images for the MIC top 5 model groups.
|
 |
 |
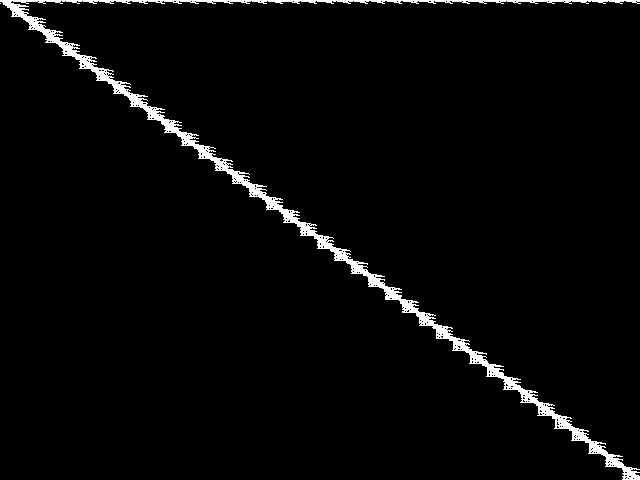 |
 |
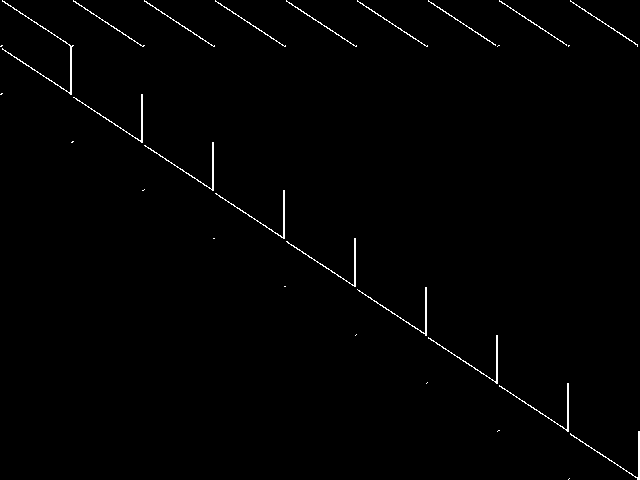 |
| Name | mapping | allcolor | graphs | fastxgemm | noip | |
|
Rank / ISS
The image-based structural similarity (ISS) metric measures the Euclidean distance between the image-based feature vectors for the query instance and all other instances. A smaller ISS value indicates greater similarity.
|
1 / 1.395 | 2 / 1.554 | 3 / 1.578 | 4 / 1.604 | 5 / 1.617 |
Model Group Summary
The table below contains summary information for the five most similar model groups to mitre according to the MIC.
| MODEL GROUP | SUBMITTER | DESCRIPTION | ISS | RANK | |
|---|---|---|---|---|---|
| MIC Top 5 | mapping | Gleb Belov | These are the models from MiniZinc Challenges 2012-2016 (see www.minizinc.org), compiled for MIP WITH INDICATOR CONSTRAINTS using the develop branch of MiniZinc and CPLEX 12.7.1 on 30 April 2017. Thus, these models can only be handled by solvers accepting indicator constraints. For models compiled with big-M/domain decomposition only, see my previous submission to MIPLIB.To recompile, create a directory MODELS, a list lst12_16.txt of the models with full paths to mzn/dzn files of each model per line, and say$> ~/install/libmzn/tests/benchmarking/mzn-test.py -l ../lst12_16.txt -slvPrf MZN-CPLEX -debug 1 -addOption "-timeout 3 -D fIndConstr=true -D fMIPdomains=false" -useJoinedName "-writeModel MODELS_IND/%s.mps" Alternatively, you can compile individual model as follows: $> mzn-cplex -v -s -G linear -output-time ../challenge_2012_2016/mznc2016_probs/zephyrus/zephyrus.mzn ../challenge_2012_2016/mznc2016_p/zephyrus/14__8__6__3.dzn -a -timeout 3 -D fIndConstr=true -D fMIPdomains=false -writeModel MODELS_IND/challenge_2012_2016mznc2016_probszephyruszephyrusmzn-challenge_2012_2016mznc2016_probszephyrus14__8__6__3dzn.mps | 1.394677 | 1 |
| allcolor | Domenico Salvagnin | Prepack optimization model. | 1.554043 | 2 | |
| graphs | Michael Bastubbe | Packing Cuts in Undirected Graphs. Models are described in 4.1. | 1.577754 | 3 | |
| fastxgemm | Laurent Sorber | Naive multiplication of two N by N matrices requires N^3 scalar multiplications. For N=2, Strassen showed that it could be done in only R=7 < 8=N^3 multiplications. For N=3, it is known that 19 <= R <= 23, and for N=4 it is known that 34 <= R <= 49. This repository contains code that generates a mixed-integer linear program (MILP) formulation of the fast matrix multiplication problem for finding solutions with R < N^3 and proving that they are optimal. For a more detailed description, see the accompanying manuscript. | 1.604180 | 4 | |
| noip | Christopher Hojny | integer programming formulation that verifies that no integer programming formulation of a given 0/1-point set exists | 1.616524 | 5 |

