×
![]()
ta1-UUM: Instance-to-Instance Comparison Results
| Type: | Instance |
| Submitter: | MIPLIB submission pool |
| Description: | Imported from the MIPLIB2010 submissions. |
| MIPLIB Entry |
Parent Instance (ta1-UUM)
All other instances below were be compared against this "query" instance.  |
 |
 |
 |
 |
 |
|
Raw
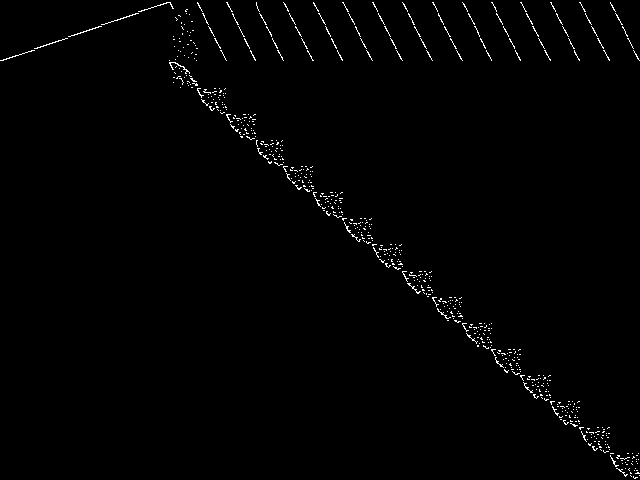
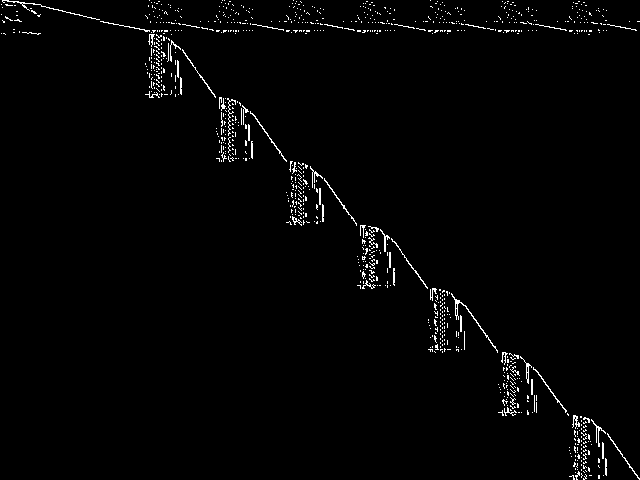
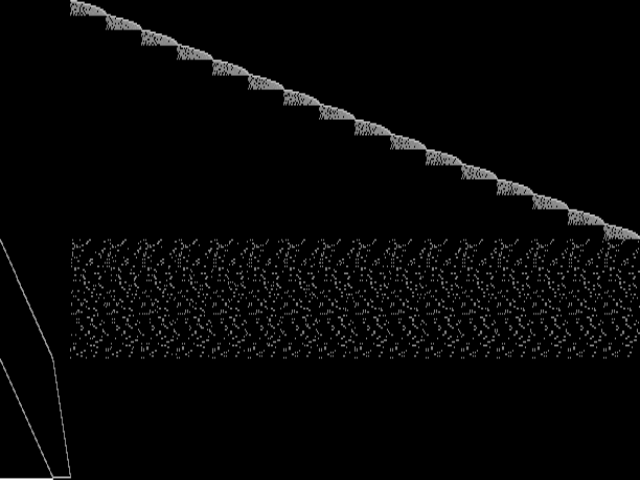
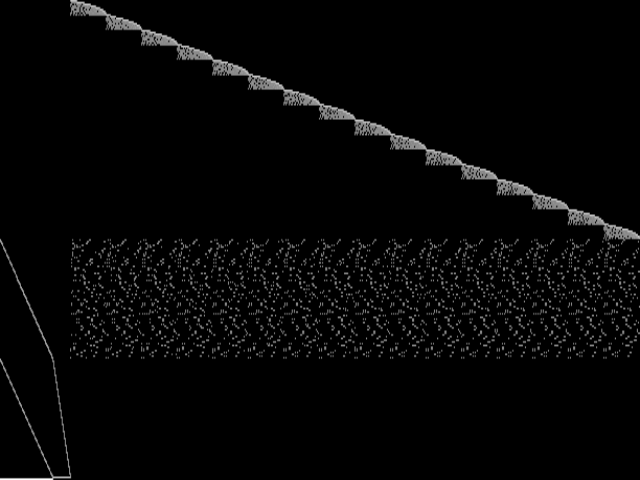
This is the CCM image before the decomposition procedure has been applied.
|
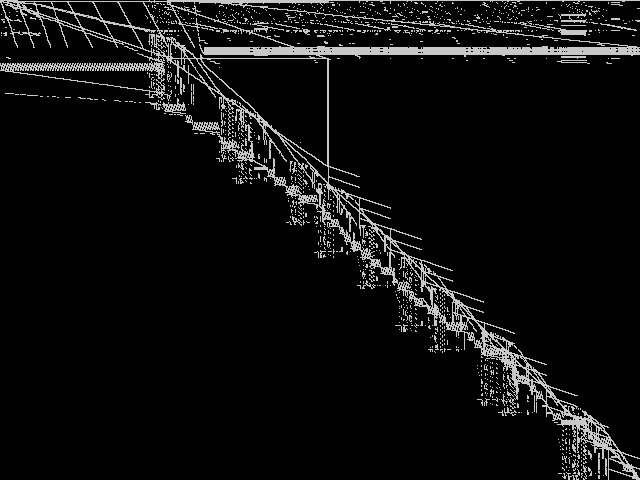
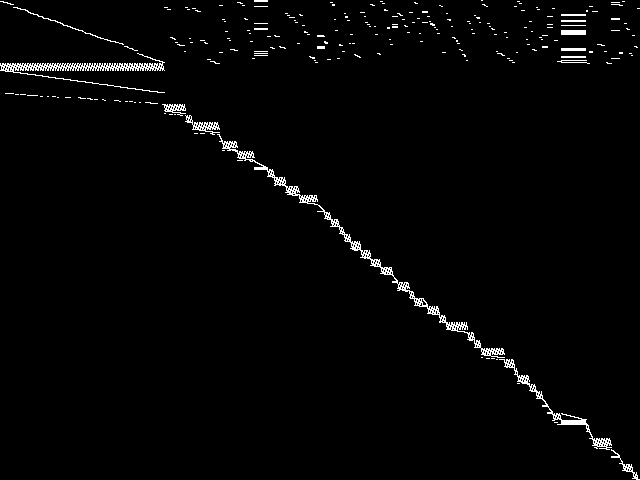
Decomposed
This is the CCM image after a decomposition procedure has been applied. This is the image used by the MIC's image-based comparisons for this query instance.
|
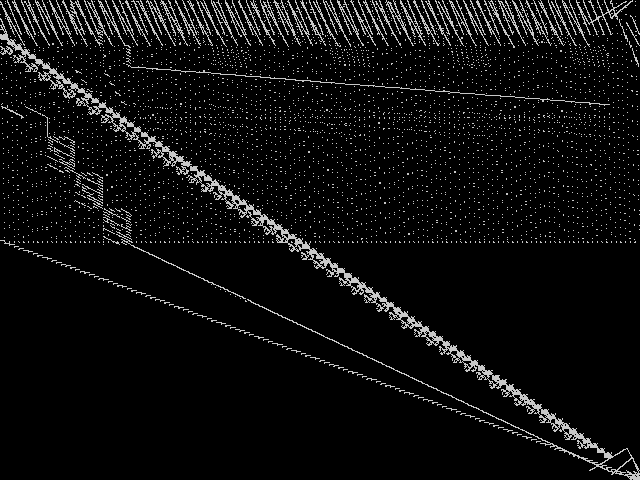
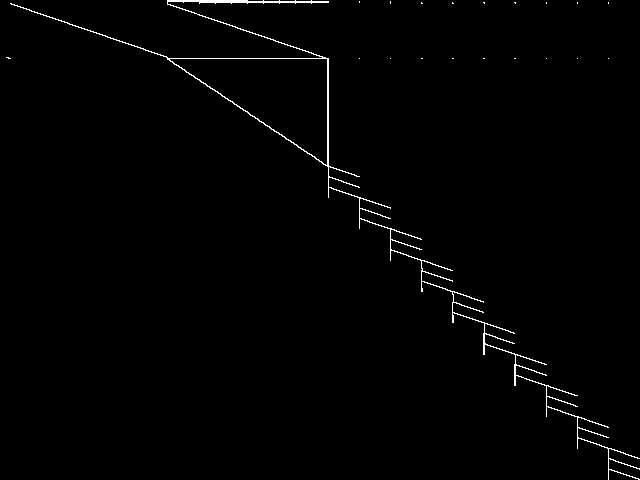
Composite of MIC Top 5
Composite of the five decomposed CCM images from the MIC Top 5.
|
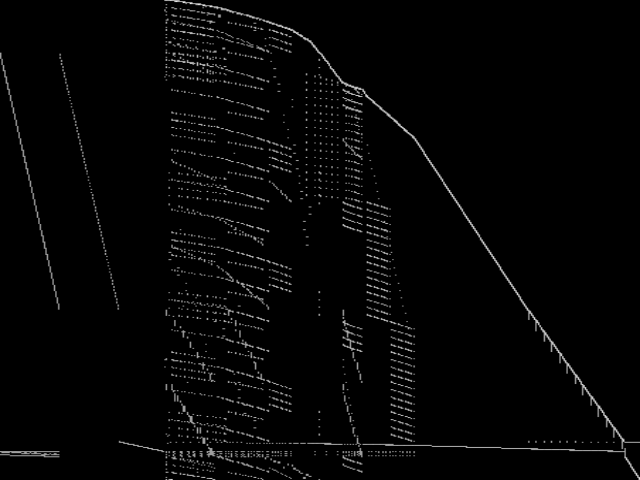
Composite of MIPLIB Top 5
Composite of the five decomposed CCM images from the MIPLIB Top 5.
|
Model Group Composite Image
Composite of the decomposed CCM images for every instance in the same model group as this query.
|
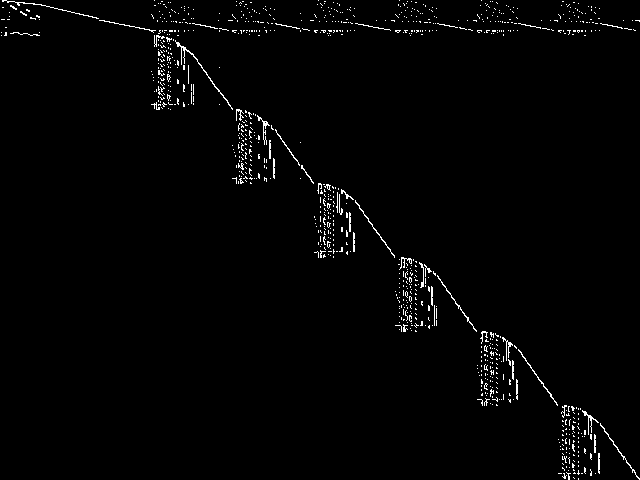
MIC Top 5 Instances
These are the 5 decomposed CCM images that are most similar to decomposed CCM image for the the query instance, according to the ISS metric.  |
Decomposed
These decomposed images were created by GCG.
|
 |
 |
 |
 |
 |
| Name | fastxgemm-n2r6s0t2 [MIPLIB] | fastxgemm-n2r7s4t1 [MIPLIB] | sct5 [MIPLIB] | mitre [MIPLIB] | neos-3615091-sutlej [MIPLIB] | |
|
Rank / ISS
The image-based structural similarity (ISS) metric measures the Euclidean distance between the image-based feature vectors for the query instance and all other instances. A smaller ISS value indicates greater similarity.
|
1 / 0.757 | 2 / 0.759 | 3 / 0.805 | 4 / 0.865 | 5 / 0.869 | |
|
Raw
These images represent the CCM images in their raw forms (before any decomposition was applied) for the MIC top 5.
|
 |
 |
 |
 |
 |
MIPLIB Top 5 Instances
These are the 5 instances that are most closely related to the query instance, according to the instance statistic-based similarity measure employed by MIPLIB 2017  |
Decomposed
These decomposed images were created by GCG.
|
 |
 |
 |
 |
 |
| Name | n9-3 [MIPLIB] | n5-3 [MIPLIB] | neos-3421095-cinca [MIPLIB] | ger50-17-ptp-pop-6t [MIPLIB] | ger50-17-ptp-pop-3t* [MIPLIB] | |
|
Rank / ISS
The image-based structural similarity (ISS) metric measures the Euclidean distance between the image-based feature vectors for the query instance and all model groups. A smaller ISS value indicates greater similarity.
|
95 / 1.186 | 228 / 1.337 | 462 / 1.541 | 531 / 1.621 | 531* / 1.621* | |
|
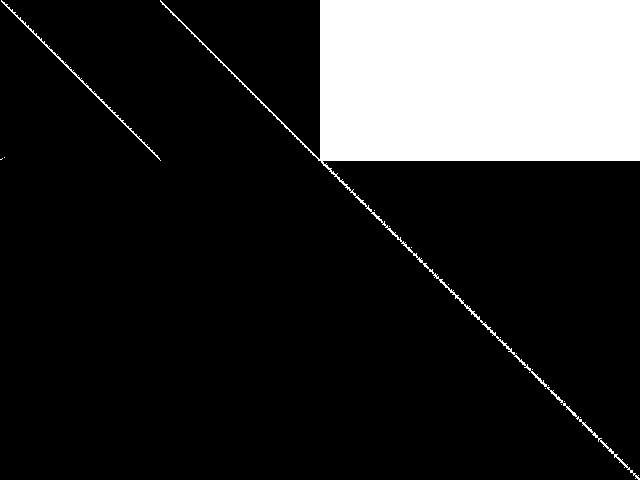
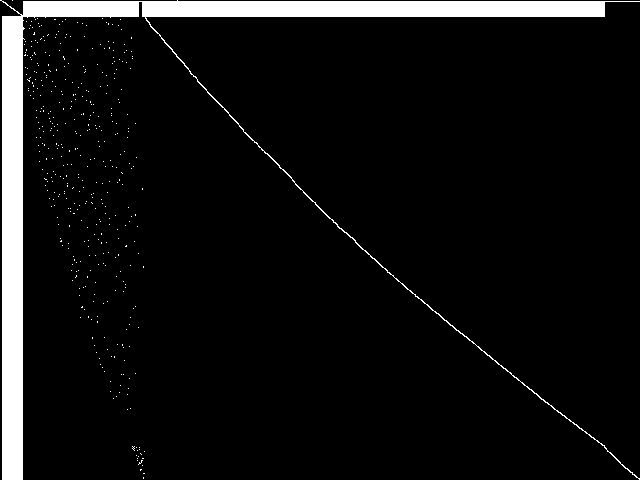
Raw
These images represent the CCM images in their raw forms (before any decomposition was applied) for the MIPLIB top 5.
|
 |
 |
 |
 |
 |
Instance Summary
The table below contains summary information for ta1-UUM, the five most similar instances to ta1-UUM according to the MIC, and the five most similar instances to ta1-UUM according to MIPLIB 2017.
| INSTANCE | SUBMITTER | DESCRIPTION | ISS | RANK | |
|---|---|---|---|---|---|
| Parent Instance | ta1-UUM [MIPLIB] | MIPLIB submission pool | Imported from the MIPLIB2010 submissions. | 0.000000 | - |
| MIC Top 5 | fastxgemm-n2r6s0t2 [MIPLIB] | Laurent Sorber | Naive multiplication of two N by N matrices requires N^3 scalar multiplications. For N=2, Strassen showed that it could be done in only R=7 < 8=N^3 multiplications. For N=3, it is known that 19 <= R <= 23, and for N=4 it is known that 34 <= R <= 49. This repository contains code that generates a mixed-integer linear program (MILP) formulation of the fast matrix multiplication problem for finding solutions with R < N^3 and proving that they are optimal. For a more detailed description, see the accompanying manuscript. | 0.756554 | 1 |
| fastxgemm-n2r7s4t1 [MIPLIB] | Laurent Sorber | Naive multiplication of two N by N matrices requires N^3 scalar multiplications. For N=2, Strassen showed that it could be done in only R=7 < 8=N^3 multiplications. For N=3, it is known that 19 <= R <= 23, and for N=4 it is known that 34 <= R <= 49. This repository contains code that generates a mixed-integer linear program (MILP) formulation of the fast matrix multiplication problem for finding solutions with R < N^3 and proving that they are optimal. For a more detailed description, see the accompanying manuscript. | 0.759254 | 2 | |
| sct5 [MIPLIB] | Siemens | Assembly line balancing for printed circuit board production | 0.804993 | 3 | |
| mitre [MIPLIB] | MIPLIB submission pool | Imported from the MIPLIB2010 submissions. | 0.864982 | 4 | |
| neos-3615091-sutlej [MIPLIB] | Hans Mittelmann | Collection of anonymous submissions to the NEOS Server for Optimization | 0.869456 | 5 | |
| MIPLIB Top 5 | n9-3 [MIPLIB] | A. Atamtürk | Capacitated network design problem | 1.185876 | 95 |
| n5-3 [MIPLIB] | A. Atamtürk | Capacitated network design problem | 1.337415 | 228 | |
| neos-3421095-cinca [MIPLIB] | Jeff Linderoth | (None provided) | 1.540787 | 462 | |
| ger50-17-ptp-pop-6t [MIPLIB] | C. Raack | Multi-layer network design problem using a link-flow formulation over a path-flow formulation. | 1.621165 | 531 | |
| ger50-17-ptp-pop-3t* [MIPLIB] | C. Raack | Multi-layer network design problem using a link-flow formulation over a path-flow formulation. | 1.621165* | 531* |
ta1-UUM: Instance-to-Model Comparison Results
| Model Group Assignment from MIPLIB: | network_design |
| Assigned Model Group Rank/ISS in the MIC: | 162 / 3.042 |
MIC Top 5 Model Groups
These are the 5 model group composite (MGC) images that are most similar to the decomposed CCM image for the query instance, according to the ISS metric.  |
These are model group composite (MGC) images for the MIC top 5 model groups.
|
 |
 |
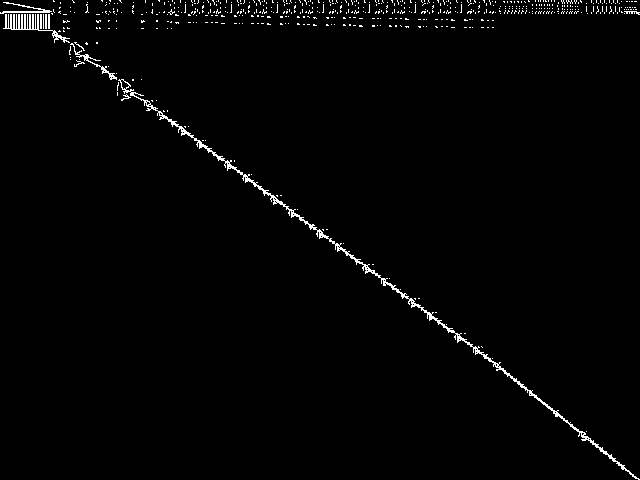 |
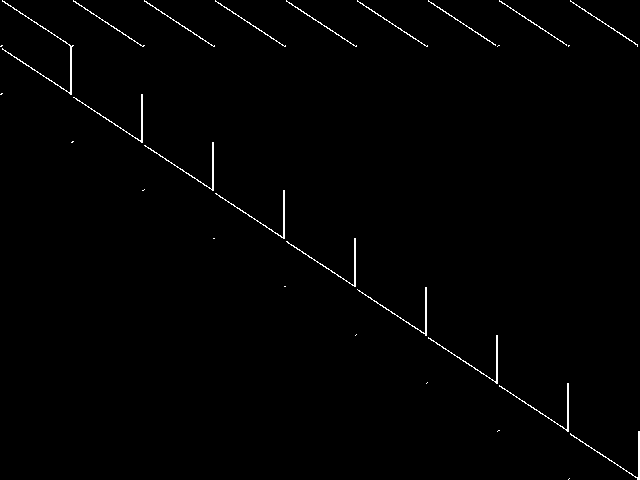 |
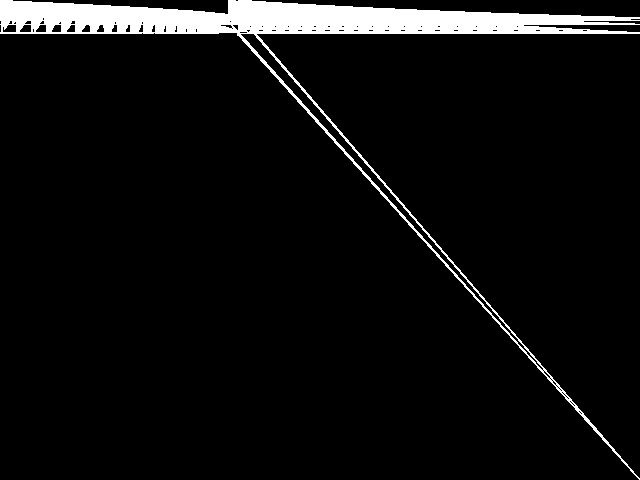 |
| Name | supportvectormachine | hypothyroid | mapping | noip | square | |
|
Rank / ISS
The image-based structural similarity (ISS) metric measures the Euclidean distance between the image-based feature vectors for the query instance and all other instances. A smaller ISS value indicates greater similarity.
|
1 / 1.529 | 2 / 1.570 | 3 / 1.616 | 4 / 1.634 | 5 / 1.655 |
Model Group Summary
The table below contains summary information for the five most similar model groups to ta1-UUM according to the MIC.
| MODEL GROUP | SUBMITTER | DESCRIPTION | ISS | RANK | |
|---|---|---|---|---|---|
| MIC Top 5 | supportvectormachine | Toni Sorrell | Suport vector machine with ramp loss. GSVM2-RL is the formulation found in Hess E. and Brooks P. (2015) paper, The Support Vector Machine and Mixed Integer Linear Programming: Ramp Loss SVM with L1-Norm Regularization | 1.528598 | 1 |
| hypothyroid | Gleb Belov | Linearized Constraint Programming models of the MiniZinc Challenges 2012-2016. I should be able to produce versions with indicator constraints supported by Gurobi and CPLEX, however don't know if you can use them and if there is a standard format. These MPS were produced by Gurobi 7.0.2 using the MiniZinc develop branch on eb536656062ca13325a96b5d0881742c7d0e3c38 | 1.570427 | 2 | |
| mapping | Gleb Belov | These are the models from MiniZinc Challenges 2012-2016 (see www.minizinc.org), compiled for MIP WITH INDICATOR CONSTRAINTS using the develop branch of MiniZinc and CPLEX 12.7.1 on 30 April 2017. Thus, these models can only be handled by solvers accepting indicator constraints. For models compiled with big-M/domain decomposition only, see my previous submission to MIPLIB.To recompile, create a directory MODELS, a list lst12_16.txt of the models with full paths to mzn/dzn files of each model per line, and say$> ~/install/libmzn/tests/benchmarking/mzn-test.py -l ../lst12_16.txt -slvPrf MZN-CPLEX -debug 1 -addOption "-timeout 3 -D fIndConstr=true -D fMIPdomains=false" -useJoinedName "-writeModel MODELS_IND/%s.mps" Alternatively, you can compile individual model as follows: $> mzn-cplex -v -s -G linear -output-time ../challenge_2012_2016/mznc2016_probs/zephyrus/zephyrus.mzn ../challenge_2012_2016/mznc2016_p/zephyrus/14__8__6__3.dzn -a -timeout 3 -D fIndConstr=true -D fMIPdomains=false -writeModel MODELS_IND/challenge_2012_2016mznc2016_probszephyruszephyrusmzn-challenge_2012_2016mznc2016_probszephyrus14__8__6__3dzn.mps | 1.615778 | 3 | |
| noip | Christopher Hojny | integer programming formulation that verifies that no integer programming formulation of a given 0/1-point set exists | 1.634173 | 4 | |
| square | Sascha Kurz | Squaring the square For a given integer n, determine the minimum number of squares in a tiling of an \\(n\\times n\\) square using using only integer sided squares of smaller size. (Although the models get quite large even for moderate n, they can be solved to optimality for all \\(n \\le 61\\), while challenging the MIP solver, especially the presolver.) | 1.655497 | 5 |

